Wikipedia:Reference desk/Archives/Mathematics/2009 February 2
| Mathematics desk | ||
|---|---|---|
| < February 1 | << Jan | February | Mar >> | February 3 > |
| Welcome to the Wikipedia Mathematics Reference Desk Archives |
|---|
| The page you are currently viewing is an archive page. While you can leave answers for any questions shown below, please ask new questions on one of the current reference desk pages. |
February 2[edit]
"Complement" of an automorphism group[edit]
I am confused as to how the notion of a "complement" (in the group-theoretic sense) can apply to non-group-elements. In the example I have, we have a quotient , and it is written "Let L- be the complement in L under the action of " where is some automorphism of Q. How can L- be defined as the complement of a group of automorphisms - what is it the complement in, and if it is how can that even be defined?? I'm lost! SetaLyas (talk) 00:34, 2 February 2009 (UTC)
- Try giving a little more context; perhaps there is some minor typo. It is not uncommon for Q to be a q-group, ρ to be an automorphism of coprime order, and to ask about a complement of the centralizer of ρ in Q/[Q,Q], which is likely equal to image of [ Q, ρ ] in L, also known as L1−ρ, another important subgroup. JackSchmidt (talk) 03:44, 2 February 2009 (UTC)
- Thanks ^_^ I'm reading from a paper filled with typos, so that's not unlikely! You're correct in some of your guesses... Q is a 2-group, is an automorphism of order (so of coprime order). It is to do with considering the associated Lie ring of the group, and then just says "Let be the complement in L under the action of ". So you are saying by "the complement in L under the action of ρ" means the complement of the centralizer of ρ (or <ρ>?) in Q/[Q,Q], which could equal [Q,ρ]/[Q,Q]? Are there any texts where this terminology is used that you know of? SetaLyas (talk) 12:27, 2 February 2009 (UTC)
- Especially if the Lie ring methods are being used to talk about fixed points or fixed point free automorphisms, I think the text means the complement of the fixed points/centralizer. I don't think leaving out the words "of the fixed points" is standard terminology, but if it is a preprint, it could be a very plausible typo. The index of the centralizer in Q of ρ should be equal to the (group theoretic, not vector space) index of L− in L (because ρ has coprime order; Khukhro's p-group book, page 81) if this is the case, so that might be something to check.
- In general if ρ acts coprimely on Q, then Q = [Q,ρ]·CQ(ρ), and if Q is abelian, then this is a direct product (so true for instance in the quotient Q/[Q,Q]). This can be found in Aschbacher's or Kurzweil and Stellmacher's or Gorenstein's group theory textbooks (I think always under "coprime action"). Again, you could check this in each factor to see if this is what the author means; it should basically just be finding the Fitting decomposition of 1−ρ.
- For Lie ring methods: Chapter VIII of Huppert-Blackburn 2 is probably reasonable place to compare to, as it covers the regular automorphism case, which is similar (especially section 9). Khukhro's p-automorphisms of finite p-groups has a reasonable description of Lie ring methods, but focused on the not-coprime case. Vaughan-Lee's book on the Restricted Burnside Problem had some good material if I recall correctly, but I don't think it focused on automorphisms at all. Leedham-Green and McKay's structure of p-groups book I think uses similar techniques to the other books, but is fairly specialized and fast paced. If the paper is online, I can probably check if this is reasonable. JackSchmidt (talk) 18:52, 2 February 2009 (UTC)
- Thanks ^_^ I'm reading from a paper filled with typos, so that's not unlikely! You're correct in some of your guesses... Q is a 2-group, is an automorphism of order (so of coprime order). It is to do with considering the associated Lie ring of the group, and then just says "Let be the complement in L under the action of ". So you are saying by "the complement in L under the action of ρ" means the complement of the centralizer of ρ (or <ρ>?) in Q/[Q,Q], which could equal [Q,ρ]/[Q,Q]? Are there any texts where this terminology is used that you know of? SetaLyas (talk) 12:27, 2 February 2009 (UTC)
mathematics[edit]
Who is the father of geometr —Preceding unsigned comment added by 74.125.74.37 (talk) 02:55, 2 February 2009 (UTC)
- In the classical western tradition, this is usually ascribed to Euclid. You may want to evaluate History of geometry to define your question more precisely, as well as consider a more world-wide perspective. Nimur (talk) 03:23, 2 February 2009 (UTC)
- y? —Preceding unsigned comment added by 82.120.227.157 (talk) 15:13, 2 February 2009 (UTC)
- Euclid is listed in Fathers of scientific fields#Mathematics which also lists fathers of some subfields of geometry. PrimeHunter (talk) 18:01, 2 February 2009 (UTC)
Name of a type of puzzle[edit]
When I was in elementary school, teachers often gave us math puzzles during lunch for whatever reason. They were the types of puzzles where you had to form a number after being presented with a set of numbers. For example, if I am given the numbers 1,2,4,5 then I would have to manipulate them in a way where the result would be 24. The answer, of course would be 4(5+2-1). Is there a name for this? Thanks, Vic93 (t/c) 04:28, 2 February 2009 (UTC)
- Your variant sounds like 24 Game. The best known variant may be four fours. I don't know whether there is a general name for this type of puzzle, but see Krypto (game). PrimeHunter (talk) 04:37, 2 February 2009 (UTC)
- Hi, thanks. I certainly didn't know about that game before. However, looking back now, I may have misworded my original query. While 24 does sound a lot like what I described, I neglected to mention that the end result could be any integer, not just 24. For example, I would be given the numbers 3,7,5,2 with the goal being say, 8. The answer would be ((7*3)-5)/2. Also, I don't believe it was limited to four numbers. You could be given three, five, thirty (although that would be extremely difficult), etc. Perhaps this is just another variant of the game, but I'd like to know if it has another name (if it is not indeed 24). Vic93 (t/c) 22:15, 2 February 2009 (UTC)
Is it possible to generate any integer, as constrained in the Four fours article (using addition, multiplication, concatenation, factorial, exponentiation)? No logs, as it states that it is trivial to do with them. Nadando (talk) 05:37, 2 February 2009 (UTC)
- You may have to allow division, subtraction, square roots and floor function as well, to get many numbers. I am guessing it is not possible to generate any integer, but stated without proof! How would you make 0 1 2 3 and other small numbers with out some operation that would produce smaller numbers. Graeme Bartlett (talk) 06:22, 2 February 2009 (UTC)
- The book Mathematical Recreations and Essays (mentioned in the article) is available on google books. Please check out page 14. Depending on what opperations are allowed you can go up to different numbers. Anythingapplied (talk) 17:13, 2 February 2009 (UTC)
What is this type of problem called?[edit]
For example, you have a container of capacity 3 and another of capacity 5, and have to use fill and transfer operations to measure out, for example, 2 units. One solution is to fill the 5 container, then transfer 3 units to fill the other container, leaving 2 units behind. My question:
(a) Does this type of problem have a name? (b) For general containers of capacity m and n units, which values of quantity can be produced, and what sequence of steps are required in each case?→86.132.164.81 (talk) 13:11, 2 February 2009 (UTC)
- (b) Values: all multiples of the greatest common divisor d of m and n, which is also characterized as the smallest positive integer of the form d=xn+ym over integers x,y; the corresponding algorithm is old Euclid's one. Not by chance: if you replace "containers & capacity" with "segments & length" the geometric origin of the problem appears. --131.114.72.215 (talk) 13:23, 2 February 2009 (UTC)
- I don't see how to implement Euclid's algorithm with two containers. If you have a units in one container, and b in the other, how do you replace a with a mod b or a − b while leaving b intact? I don't think you can produce gcd(m, n) in general without using emptying a container as another operation (and even in that case the algorithm is not Euclid's, but a sort of "counting x times n modulo m" for a suitable x). Furthermore, you obviously cannot fit more than m + n units in containers of size m and n, so arbitrary multiples of the gcd are out of question. — Emil J. 14:14, 2 February 2009 (UTC)
- Example: let m = 5 and n = 7. If we denote by (a, b) the state where a units are in the smaller container and b units in the larger, then it is easy to see that the following set of states in closed under the operations of filling and transfering: (0, 0), (5, 0), (0, 7), (5, 7), (0, 5), (5, 2). Thus you cannot produce gcd(5, 7) = 1. — Emil J. 14:31, 2 February 2009 (UTC)
- Yes, I was just wondering whether to add a remark... If you are allowed to use only the two containers, filling and emptying them (e.g. you are at the sea), then of course you get exactly all multiples of the gcd up to n+m (and potentially any multiple if e.g. you drink it). If you impose the constraint that you can't waste water, then it is your situation (and the answer is different as you say). The OP refers to "fill" and "transfer" operations indeed (86: is unfill=-fill allowed??). But your ecological version is somehow more attractive. --pma (talk) 14:44, 2 February 2009 (UTC)
- I believe this is called the Die Hard with a Vengeance problem. -mattbuck (Talk) 15:35, 2 February 2009 (UTC)
- Fill 7; Pour 7 into 5 leaving 2; Throw 5 away; Pour 2 in 7 into 5; Fill 7; Pour 3 of 7 into 5 leaving 4 in 7; Throw 5; Pour 4 in 7 into 5; Fill 7; Pour 1 of 7 into 5 leaving 6 in 7; Throw 5; Pour 5 of 6 in 7 into 5 leaving 1 in 7; Throw 5. You have 1 in 7. -- SGBailey (talk) 20:47, 2 February 2009 (UTC)
If you were allowed negative amounts, I think the greatest common divisor answer would be right. Michael Hardy (talk) 01:36, 3 February 2009 (UTC)
Expanding an integral[edit]
I am trying to expand the following expression for small ξ:
Just expanding the integrand and integrating term by term does not work, since it runs into ever more divergent integrals. I guess the expansion will involve log terms and the like... Does anybody have an idea of how to do it? Thanks, MuDavid ![]() 15:00, 2 February 2009 (UTC)
15:00, 2 February 2009 (UTC)
- Ok, I found it myself:
- MuDavid
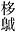 10:29, 3 February 2009 (UTC)
10:29, 3 February 2009 (UTC)
- I followed a rather sinuous path. In fact I was calculating the analytic continuation of
- for d = 3. Summing first and taking the integrals together gives π–2 times the integral I wrote above. If, on the other hand, you integrate first (for suitable values of d sum and integral can be switched at will), you get
- (The integrals may look suspicious, but some juggling with analytic continuations gives the right result. These expressions can be found in about any textbook on quantum field theory.) Now you set the term with n = 0 apart (this one will give the ξ3 term), and you rewrite the remainder of the sum as going from one to infinity. Then you expand in ξ. For suitable values of d, the summation over n can be switched with the sum of the expansion. Performing the sum over n gives Riemann zeta functions. Then you take the limit d to 3. One of the terms in the expansion has ζ(1), while the second piece (without the sum) has Γ(–2). The poles cancel exactly and a term with ln(ξ) emerges as a second-order term in the expansion of ξd+1.
- I have two more expression like that. They are more complicated, but the procedure can be readily applied (albeit less beautifully; I don't have a closed expression for the general term). MuDavid
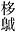 08:45, 4 February 2009 (UTC)
08:45, 4 February 2009 (UTC)
- I followed a rather sinuous path. In fact I was calculating the analytic continuation of
- ;-) Well, I do quantum field theory all day long, so this "finding an expression in any textbook about quantum field theory" is not much of a feat, really. I'm proud of the Riemann zeta functions, though. MuDavid
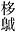 13:40, 4 February 2009 (UTC)
13:40, 4 February 2009 (UTC)
- ;-) Well, I do quantum field theory all day long, so this "finding an expression in any textbook about quantum field theory" is not much of a feat, really. I'm proud of the Riemann zeta functions, though. MuDavid
Capitalization conundrum[edit]
Hello all. I'm writing because my office mate and I were having a discussion concerning the capitalization of terms in mathematics that use a person's name. In particular, we're concerned with those names that get turned into adjectives. We're thinking "Boolean", "Abelian", "Cauchy", "Lipschitz", things like that. (For instance, a function can be Lipschitz, but no one would ever say "the graph is Petersen").
We noticed that almost everyone gets their name capitalized except for Abel. The word "abelian" appears in lowercase all over the place. What gives? Can anyone explain this to us? Also, does anyone have other examples of lowercase typeset names?
Thanks! –King Bee (τ • γ) 17:42, 2 February 2009 (UTC)
- I always think of it as it being an extra honour, not getting a capital letter (a bit like members of the Royal College of Surgeons going by Mr. not Dr.). Abel is such a great mathematician that something named after him has being a word it its own right and is no longer thought of as eponymous. (Take a group of students that have just finished a first year algebra course and see how many of them even know where the term "abelian" comes from!) --Tango (talk) 18:09, 2 February 2009 (UTC)
- The late professor Børge Jessen said that the highest honor for a mathematician is to become an adjective spelled without capitalization. He mentioned, apart from abelian, also galois groups, and hermitean and pythagorean and euclidean. I think he also wrote 'hilbert space'. Bo Jacoby (talk) 18:16, 2 February 2009 (UTC).
- I agree with Tango (although the honor is slightly diluted by having an exact synonym -- commutative -- in common use), but I also never capitalize boolean (largely because of programming). I don't have any explanation for why these conventions are used, though... maybe "Abel" and "Boole" just don't sound like typical western last names? By the way, the term for being names after something is eponym. Eric. 131.215.158.184 (talk) 18:50, 2 February 2009 (UTC)
- It's also worth noting your sample set there was quite strange. Lipschitz and Cauchy will always be capitalised, as they are the surnames themselves, not adjectives derived from them. Adjectives derived from names are uncapitalised through increased usage, there is no "correct" way... SetaLyas (talk) 21:22, 2 February 2009 (UTC)
- Compare to the standardized metric system notation, "Symbols for units are written in lower case, except for symbols derived from the name of a person. For example, the unit of pressure is named after Blaise Pascal, so its symbol is written "Pa", whereas the unit itself is written "pascal"." Nimur (talk) 09:18, 3 February 2009 (UTC)
- SetaLyas - Sorry. Replace Lipschitz and Cauchy with Gaussian and Hermitian.
- The Rest - Thanks for your input. –King Bee (τ • γ) 13:54, 3 February 2009 (UTC)
Lots of people who write Wikipedia articles don't capitalize "boolean", and since many of those are in computer science, I wonder if they know "Boole" is a person's name (in computer science it's compulsory to start every word with a capital letter except when there's a reason to do so). I've noticed people not capitalizing "gaussian" and I wonder if they know that Gauss was the most famous person to live on earth in the 19th century (except among those who did not work in the physical and mathematical sciences). Michael Hardy (talk) 15:14, 3 February 2009 (UTC)
- Isn't platonic and christian written without capitals, even if people do know that Platon and Christ are famous people? Bo Jacoby (talk) 11:53, 4 February 2009 (UTC).
- In English it isn't, is it? — Emil J. 16:36, 4 February 2009 (UTC)
- I would certainly write Christian with a capital C. Platonic, I'm not so sure about... Incidentally, isn't it Plato, not Platon? --Tango (talk) 22:48, 4 February 2009 (UTC)
- Plato is the usual English version, but Platon is a direct transliteration of (the nominative form of) his name in Greek, and I believe it's the standard form in some modern languages. Algebraist 22:51, 4 February 2009 (UTC)
Golden ratio tesselation?[edit]
<img src="http://www.cantonese.ca/quilt-model.png"> I want to adjust the ratios and angles in this tesselation to make the square bigger, possibly into a golden rectangle, and adjust the rhombi accordingly. Can anyone work out what dimensions and angles to use for it to "work" mathematically? --Sonjaaa (talk) 20:14, 2 February 2009 (UTC)
What ratio? All the edges are the same length. All you can do is alter the angle between the squares. At one extreme (0 degrees) the rhombii will cease to exist and you'll have tessellating squares at the other extreme (90 degrees) the rhombii will become more squares so you'll have tessellating squares again. An obvious compromise is to use 45 degrees. -- SGBailey (talk) 20:52, 2 February 2009 (UTC)
Is it possible to turn the square into a rectangle, a golden rectangle, and adjust the rhombi accordingly? Or would that be geometrically impossible?--Sonjaaa (talk) 21:42, 2 February 2009 (UTC)
Hi Sonjaaa... yes you can do the same with any rectangle, also a golden one, in place of the red squares, the only thing is that the pink and magenta rhombi will not be equal. You can decide both edges of the rectangle, say thy are a and b. Then you also have all rhombi of one color with all edges =a, of course, and rhombi of the other color with all edges =b. The rectangles of course has 90 degree angles, the pink and magenta rhombi are similar, all of them have 2 angles of degrees, and the other 2 of degrees, and you can also decide what is . You may think that there are only the red rectangles and that they are only joined by the corners, like in your picture, and that the rhombi are just background. As SGBailey suggests, you can move all rectangles together, making the angle more or less open. When it's 90 degrees too, you get a figure like a Scottish design, if you know what I mean. When it vanises, or when it becomes 180 degrees, also, the rectangles join together like bricks. You can make it with a deck of cards. Was it as simple as that what you meant, or do you want another thing? Also, if you take a graph paper you may draw it easily :) pma (talk) 00:11, 3 February 2009 (UTC)
- You could try other tessellations, or go with Penrose tiling for instance if you really want to make bigger and bigger patterns. Dmcq (talk) 09:31, 3 February 2009 (UTC)
- If I change the square to a golden rectangle with dimensions 1000 by 1618, then what would be the dimensions and angles of the rhombi? I want to cut out a pattern I can use for a quilt, but I don't know how to get the exact measurements for the rhombi except by trial and error until something fits at the right angles.--Sonjaaa (talk) 17:41, 3 February 2009 (UTC)
- Oh I see, it's like an algebra puzzle and you gave me the formula with the alpha thingy.--Sonjaaa (talk) 17:42, 3 February 2009 (UTC)
- Sonjaaa, since you still have to fix the shape of the rhombi, if you want to be super-golden, why don't you choose golden rhombi too? That is, they have the longer diagonal and the shorter diagonal in golden ratio (all rhombi, small and large). Dimensions:
- Rectangle's Shorter Edge: 1000
- Rectangle's Longer Edge: 1618
- Small Rhombus' Edge: 1000
- Small Rhombus' Shorter Diagonal: 1051
- Small Rhombus' Longer Diagonal: 1701
- Large Rhombus' Edge: 1618
- Large Rhombus' Shorter Diagonal: 1701
- Large Rhombus' Longer Diagonal: 2753
- This choice also makes the small rhombus' longer diagonal equal to the large rhombus' shorter diagonal (appreciate the Chiasmus), that makes the whole thing veeery harmonious --you know... ;) I didn't wrote angles because the diagonals are enough, to draw a rhombus (it's easier and more precise). Do you like the hint? pma (talk) 20:48, 3 February 2009 (UTC)
- Sonjaaa, since you still have to fix the shape of the rhombi, if you want to be super-golden, why don't you choose golden rhombi too? That is, they have the longer diagonal and the shorter diagonal in golden ratio (all rhombi, small and large). Dimensions:
- Oh I see, it's like an algebra puzzle and you gave me the formula with the alpha thingy.--Sonjaaa (talk) 17:42, 3 February 2009 (UTC)
Function proof, hard one[edit]
g(g(k)+g(m))=k+m.
k,m are greater or equal to zero, g(x) has x greater or equal to 0, and g(x) is greater or equal to 0.
(all defined to be greater or equal to zero)
Prove or Disprove:
g(x)=c (with restrictions for greater or equal to 0, constant c) and g(x)=x (with restrictions) are the ONLY TWO FUNCTIONS that satisfy this.
Its easy to see that they satisfy.
if g(x)=x, then g(g(k)+g(m))=k+m. because g(g(k)+g(m))=g(k+m)=k+m if g(x) =x, the left side is obviously k+m so they satisfy...
its also easy to tell for constant c.
However, HOW DO YOU FIND OTHER FUNCTIONS!?/ (like exponential, log ones)
Thanks —Preceding unsigned comment added by 208.119.135.108 (talk) 21:00, 2 February 2009 (UTC)
- I don't understand. If g(x)=c, then g(g(k)+g(m))=c, which is not necessarily equal to k+m, surely? Algebraist 21:03, 2 February 2009 (UTC)
- But K+M=constant (since they are both integers) so it is a constant. —Preceding unsigned comment added by 208.119.135.108 (talk) 22:14, 2 February 2009 (UTC)
- Oh, are k and m fixed integers? I assumed they were variable (nonnegative) reals. In that case, there are enormous numbers of such functions. Since the given condition only involves the value of g at three points (k, m and g(k)+g(m)), you can choose g freely at all other points and still get a function satisfying the condition. Algebraist 22:18, 2 February 2009 (UTC)
- But K+M=constant (since they are both integers) so it is a constant. —Preceding unsigned comment added by 208.119.135.108 (talk) 22:14, 2 February 2009 (UTC)
see, well i think g has to be degree less than 1, because g(g(x)) is degree m lets say, well m+k is degree 1...g(g(x)) cannot be degree one if g(x) is greater than degree one
anybody know how to best appraoch this? —Preceding unsigned comment added by 208.119.135.108 (talk) 22:24, 2 February 2009 (UTC)
- What is this 'degree' you're talking about? I've already given you many solutions. Here's one particular one: set g(m)=m, g(k)=k, g(m+k)=m+k and g(x)=ex for all other values of x. Algebraist 22:27, 2 February 2009 (UTC)
- Polynomial degree? If you require that the function be a polynomial then your observations about degree is a proof, just consider what properties are needed for a first degree polynomial to satisfy g(g(k)+g(m))=k+m. I have no idea if g(x)=x and g(x)=c=k+m are the only analytic functions, and if you allow general functions then, as Algebraist notes, counterexamples are easy to construct. Taemyr (talk) 23:18, 2 February 2009 (UTC)
- Never mind. This is bullshit. Even if you limit yourself to polynomials you get counterexamples. For example, three points define a second degree polynomial. For higher degrees than that you are underspecified and get infite functions. Taemyr (talk) 23:22, 2 February 2009 (UTC)
- Yes, there are lots of polynomial solutions. The only question is which of them are everywhere nonnegative (as required by the question). This will depend on the values of k and m. Algebraist 23:23, 2 February 2009 (UTC)
- Never mind. This is bullshit. Even if you limit yourself to polynomials you get counterexamples. For example, three points define a second degree polynomial. For higher degrees than that you are underspecified and get infite functions. Taemyr (talk) 23:22, 2 February 2009 (UTC)
- (edit conflict) I think Algebraist is getting a bit annoyed. You can change the g(x)=ex to anything in the above solution and it will work. Algebraist has said this answer based on the fact that you said K and M are CONSTANTS, which I don't believe is what you meant. They probably should be variable integers. When you say g(x)=c is a solution, that implies g(x)= a constant should work no matter what constant. so g(x)=2 should work. Clearly this does not work unless k+m=2. So it only works on a very specific set of k, m. If I saw this problem I would assume that for a given function g(x) you should get g(g(k)+g(m))=k+m for ALL positive integers k and m. That being said, I think algebraist is right that the way you've purposed this problem, g(x)=c is not a solution.
- Polynomial degree? If you require that the function be a polynomial then your observations about degree is a proof, just consider what properties are needed for a first degree polynomial to satisfy g(g(k)+g(m))=k+m. I have no idea if g(x)=x and g(x)=c=k+m are the only analytic functions, and if you allow general functions then, as Algebraist notes, counterexamples are easy to construct. Taemyr (talk) 23:18, 2 February 2009 (UTC)
- Now that that is hopefully understood, let me try and lend a hand. First of all a proof that g(0)=0. Suppose g(0)=x for some integer x not equal to 0. Then by the property 0+0=g(g(0)+g(0))=g(x+x)=g(2x). So g(2x)=0. Thus by the property 2x+2x=g(g(2x)+g(2x))=g(0+0)=g(0). So g(0)=4x, which is a contradiction since g(0)=x. Thus g(0)=0 for all g(x) that abbide by the property (another reason why g(x)=c is not a solution).
- Take g(1)=x for some integer x. By the property (and prior proof)
- 1+0=g(x+0)=g(x)
- 1+1=g(x+x)=g(2x)
- x+0=g(1+0)=g(1)
- x+x=g(1+1)=g(2)
- 2x+x=g(2+1)=g(3)
- 2x+2x=g(2+2)=g(4)
- 2+1=g(2x+1x)=g(3x)
- 2+2=g(2x+2x)=g(4x)
- You can see that if we continue in this way we can show that g(kx)=k for any k and g(k)=kx for any k. I think this shows that the only possible value for x is 1 (I'm trying to think why, but am running out of time). And using this value of x=1 we've shown g(x)=x. Thus this is the only solution. Anythingapplied (talk) 23:39, 2 February 2009 (UTC)
- You must have x=1, since you have g(x)=1 and g(x)=x2. If we take everything to range over the nonnegative reals (rather than integers), then g(x)=x is still the only solution, but the proof is somewhat more effort. Algebraist 23:52, 2 February 2009 (UTC)
- If you have proven that g(x)=x is the only solution that works for the domain of the non negative integers you have also proven that no function other than g(x)=x works for the domain of nonnegative reals. Taemyr (talk) 01:31, 3 February 2009 (UTC)
- How so? Algebraist 01:40, 3 February 2009 (UTC)
- If you have proven that g(x)=x is the only solution that works for the domain of the non negative integers you have also proven that no function other than g(x)=x works for the domain of nonnegative reals. Taemyr (talk) 01:31, 3 February 2009 (UTC)
- You must have x=1, since you have g(x)=1 and g(x)=x2. If we take everything to range over the nonnegative reals (rather than integers), then g(x)=x is still the only solution, but the proof is somewhat more effort. Algebraist 23:52, 2 February 2009 (UTC)
- You can see that if we continue in this way we can show that g(kx)=k for any k and g(k)=kx for any k. I think this shows that the only possible value for x is 1 (I'm trying to think why, but am running out of time). And using this value of x=1 we've shown g(x)=x. Thus this is the only solution. Anythingapplied (talk) 23:39, 2 February 2009 (UTC)
- I don't think this question is appropriate for the reference desk. This problem is on the USAMTS, a mathematical talent search based on the honor system that gives you a month to solve problems like these. [1]. The answers to these questions should not be given until after March 9th. Indeed123 (talk) 02:11, 3 February 2009 (UTC)
- Well, at least now we know the actual question. I think the real case is more interesting, though. Algebraist 02:21, 3 February 2009 (UTC)

![{\displaystyle L=Q/[Q,Q]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2db55785d99e85f0292feb0240412e9aee078477)











